Beam Deflection Calculator
No bracket will remain perfectly straight under even the lightest load. Even if a feather were placed on a 1/2” thick steel plate, the plate would be effected by the weight. The bending in this instance would, however, be too small to notice and the steel plate certainly wouldn't be in any danger of failing.
This document uses engineering beam theory to predict the deflection of a steel plate under a given amount of weight. This is not intended to be a perfect representation of the behavior of a bracket under a load. It is only a tool to help you get an “idea.” There are several assumptions and simplifications that have been applied. Whenever possible we tried to make these assumptions as a “worst case scenario.”
Assumptions:
1) The bracket will be supporting the entire weight of the countertop. In most applications, the countertop is overhung, with the main body of granite being supported by a knee wall or cabinet. When this is the case, the rigidity of the granite provides extra support so that the bracket is only supporting a fraction of the overhung weight.
2) The bracket is ungusseted. A gusset adds significant strength to a bracket, but makes the calculations much more complex. This calculator is not a good indicator of the strength of a gusseted bracket.
3) The bracket has no holes in the top. Holes weaken the bracket slightly but this is nearly always a negligible difference.
Simplifications:
1) The bracket is treated as a cantilevered beam. The difference between a bracket and a cantilevered beam is shown below. Because of this simplification, this calculator does not account for any bending that may take place on the vertical leg.
This document uses engineering beam theory to predict the deflection of a steel plate under a given amount of weight. This is not intended to be a perfect representation of the behavior of a bracket under a load. It is only a tool to help you get an “idea.” There are several assumptions and simplifications that have been applied. Whenever possible we tried to make these assumptions as a “worst case scenario.”
Assumptions:
1) The bracket will be supporting the entire weight of the countertop. In most applications, the countertop is overhung, with the main body of granite being supported by a knee wall or cabinet. When this is the case, the rigidity of the granite provides extra support so that the bracket is only supporting a fraction of the overhung weight.
2) The bracket is ungusseted. A gusset adds significant strength to a bracket, but makes the calculations much more complex. This calculator is not a good indicator of the strength of a gusseted bracket.
3) The bracket has no holes in the top. Holes weaken the bracket slightly but this is nearly always a negligible difference.
Simplifications:
1) The bracket is treated as a cantilevered beam. The difference between a bracket and a cantilevered beam is shown below. Because of this simplification, this calculator does not account for any bending that may take place on the vertical leg.
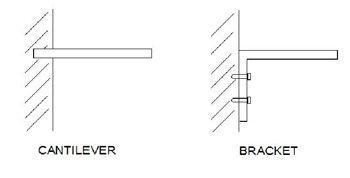
Plate Deflection Calculation Explanation:
The 5 considerations listed below are applied to the deflection calculations:
1) Material. The type of plate material will impact the deflection due to differences in desities.
2) Load. This is the amount of weight the steel plate will be supporting.
3) Length. Length of the plate.
4) Width. Width of the steel plate.
5) Thickness. Thickness of the plate material. A thicker metal plate is much better at supporting heavier loads.
Deflection
The deflection indicates how far the furthest edge of the steel plate will go down under the applied weight. There are 2 deflections calculated, depending on where the weight is:
The deflection indicates how far the furthest edge of the steel plate will go down under the applied weight. There are 2 deflections calculated, depending on where the weight is:
1) Distributed load. This is the value that is of concern in most applications. This is when the weight (entered in “Load” above) is spread out over the entire length of the bracket.
2) Point load at end. This assumes that all of the weight (entered in “Load” above) is at the very tip of the bracket.
Plate Deflection Equations:
The equations used in deflection calculations are given below.
- Distributed load deflection =
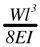
- Point load deflection =
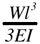
Where
E= Modulus of Elasticity (in pounds per square inch)
I= Moment of Inertia (in inches^4)
W= Load (in pounds)
l= The length of the bracket (in inches)
